Money and management decisions: When to spend (Proceedings)

Most farmers and ranchers do not have an endless supply of money with which to run their business each year. Operational decisions should be made based on expenditures that offer a monetary benefit that exceeds its cost of implementation. Analytic techniques that assist a short-term (≤1 year) operational decision-making perspective include partial budgeting, decision trees, and standardized performance analysis (SPA).

Partial Budgeting

Partial budgeting is a tool that helps estimate the effect that the allocation of money to a management change for a current program will have on profitability to the farm or ranch operation. When developing a partial budget, only the revenue and costs affected by the specific change are considered. Consequently, this technique examines only a small part of business activities. If the proposed management change will affect the entire farm or ranch enterprise, a partial budget is inappropriate to use. Instead, a total enterprise budget is needed based upon a full cost analysis in which all variable and fixed costs are considered.

Typically, a partial budget assumes that the outcome of the analysis will occur exactly as calculated; thus variability in inputs and subsequently their effect on output of results is not considered. A partial budget is divided into two sections: added returns and added costs.Added returns include (1) the increased revenue generated by the proposed management change and (2) decreased costs resulting from the proposed management change. Added costs include (1) the increased costs associated with the implementation of the proposed management change and (2) the amount of revenue foregone because of the proposed management change. The difference between added returns and added costs determines if the proposed management change is more profitable (>$0) or less profitable (<$0) than the status quo. Only profitable management changes should be considered for implementation.

The following scenario is presented to illustrate the partial budget concept: A beef cow-calf rancher comes to your veterinary clinic during the early summer to enlist your help in determining why over the past few years he has averaged 12 bred cows of various ages (out of 200 breeding cows) that are thinner than herdmates heading into the winter feeding period. In addition to their winter pasture, he can usually get some back in shape after segregating and feeding them 5 lb of 20% protein cubes per head daily for 90 days. The feed cost ($300/ton; $0.15/lb) and associated labor to feed and care for them ($10/hr; 0.50 hr) are estimated to be $1.167 per cow per day ([5 lb X $0.15/lb] + [($10/hr X 0.50 hr) / 12 hd]). At the end of this feeding period, 6 cows typically remain thin and are culled because they do not breed back after calving. He buys young bred cows to replace these cull cows. He sold these cull cows last year at a loss of $675 ($1100 replacement cost and $425 salvage value per head) and expects these market prices to remain steady for the next couple years.

After a visit to the ranch and a thorough investigation of all the factors that can be responsible for a "thin cow" problem, internal parasitism is determined to be the root cause. You recommend that this producer institute a deworming program for all his cows this winter and again sometime during the spring months before turnout to grazing pastures. You believe this should essentially alleviate the reproductive failure observed in these problem "thin cows" and the necessity to cull them. Following anthelmintic treatment, body condition should improve enough to restore reproductive efficiency in these cows to that of the rest of the herd as well as follow their same culling pattern. His current weaned calf crop is 84% (520 lb average steer calf weaning weight; 500 lb average heifer weaning weight). He routinely keeps back around 26% (22 Hd) of the weaned heifers as replacements in order to keep his cow herd at a stable size (he shows you records that around 89% (178 Hd) of the cow herd calves each year). The owner is willing to spend the $5.50 per head (anthelmintic, labor, miscellaneous) to deworm the cow herd. A 20-lb increase in calf weaning weight will also likely be realized, presumably because the cows milk better after eliminating their internal parasite burden. The producer predicts that he will sell his steer calves next fall at $108/hunderweight (cwt) base price with a $8/cwt price slide. The heifer calves will likely sell at $103/cwt along with the same price slide as steers.

Given the aforementioned information, the partial budget is constructed as follows (see Table 1).

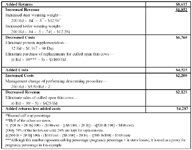
Table 1

Because the difference between added returns and added costs is a positive number (>$0), anthelmintic treatment should be implemented. The benefit to cost of this proposed short-term management change is 1.99 ($8,612/$4,325), i.e. nearly $2 is returned for every $1 allocated to the cost of this management practice.

Partial budgeting is not the only method which provides information on the benefit to cost of a proposed management change. A Standardized Performance Analysis (SPA) of the producer's cow-calf operation can also be used for this purpose.

Standardized Performance Analysis (SPA)

SPA is an economic analysis tool and not an accounting or record keeping system. It focuses on financial/economic and production performance to determine the unit cost of production (UCP), which is the cost incurred over time (up through weaning) to produce each hundredweight (cwt) of calf. Once UCP is determined, the benefit to cost of management decisions can easily and confidently be answered (e.g., "Should steer calves receive a growth promotant implant?"; "Should calves be dewormed?"). Using UCP for determining the benefit to cost of any short-term health management practices is far superior to a partial budget.

An SPA analysis is based on historical data. This includes cattle and feed inventories, accounting data required for IRS tax filings, a depreciation schedule, and accrual adjusted income statements. The SPA program is a computerized Windows-based system (Microsoft Corporation, Redmond, Wash.) that is divided into two parts: SPA production (breeding season to weaning) and SPA financial (for fiscal year calves are weaned). Numerous paper-based worksheets have been developed that can be used to help organize the data before entry into this computerized system.

Production Section

Every SPA analysis starts with the production section of the analysis. The following production data are necessary to successfully complete this section:

• General ranch and herd descriptive data

• Cowherd management/production season

• Weaned calf production and value

• Cull or breeding cattle sales

• Owned and leased land use

• Raised and purchased feed use and inventory

• Purchased and raised breeding cattle inventories

• Number of breeding females "exposed" (i.e., the original number of females turned out with bulls at the start of the breeding season)

These production data are then organized into the following categories for analysis:

• Reproduction

• Production

• Marketing description

• Grazing and raised feed

• Amount of raised and purchased feed fed

Financial Section

A producer's accountant can be of great assistance in preparing the necessary financial data used in SPA financial. The following data are necessary for the fiscal or tax year that calves are weaned in order to successfully complete the financial component of SPA:

IRS tax schedules for the fiscal year of analysis

• Depreciation schedule for the producer's farm or ranch

• Loan payment schedules from each lender

• Beginning and ending balance sheet (BS) showing all business assets, liabilities, and owner's equity

• Income statement (IS)

SPA Financial and Economic Performance Summary (cost and fair market value) includes the following:

• Investment per breeding cow (average asset values)

o Total investment per breeding cow

o Debt per breeding cow (enterprise liabilities)

o Equity-to-asset or percent equity

• Financial and economic performances

o Total raised/purchased feed cost

o Total grazing cost

o Gross cow-calf enterprise accrual-basis revenue

o Total financing cost and economic return

o Total cost before noncalf revenue adjustment (i.e., revenue adjusted for cull cow and bull sales)

o Net income

o Return on assets (ROA) percentage

• Unit cost of weaned calf production (UCP)

Further information about SPA can be found on the Texas A&M University Extension Agricultural Economics website at http://agrisk.tamu.edu.

Decision Tree Analysis

A decision tree is a method of choice for making an informed decision about how to manage an animal health problem when several options are feasible, but each one has an uncertain outcome. Thus this decision-making technique is not focused on the prediction of an event but rather on weighing the outcome of the respective options in a relative sense. The preferred action is chosen based on a predetermined decision criterion (e.g., highest expected monetary value or minimal losses).

A decision tree is constructed using lines (referred to as branches) that connect to squares (decision node), circles (chance node), and triangles (final outcome node). As the name implies, a decision tree always begin with a decision node. Leading away from it in a rightward direction are two or more branches that comprise the various decisions to be made (e.g., medical treatment vs. surgical treatment vs. salvage). The decisions must be exhaustive (i.e., include all choices to be considered) and exclusive (i.e., only one choice is ultimately possible at a decision node). Furthermore, each branch emanating away from a decision node can lead to one of three places: (1) to another decision node, which will then have two or more additional branches, representing decisions to be made, leading away from it; (2) to a chance node, which will then have two or more branches leading away from it representing potential outcomes (e.g., successful vs. unsuccessful; favorable vs. unfavorable); or (3) directly to a final outcome node (e.g. cull the animal). Likewise, branches off a chance node can lead to one of three places: to another decision node, to another chance node, or directly to an outcome. Associated with each branch emanating from a chance node is a probability that that specific outcome will occur. Mathematically, the probabilities are assigned to each branch in such a manner that they add up to 1. These probabilities can be established based on personal experience, expert opinion, unpublished experimental or clinical data, and/or published literature.

Each branch of a decision tree will eventually end with a terminal node that signifies a final outcome. Each final outcome is expressed in monetary terms. Each monetary outcome takes into account (1) the cost associated with implementing each intervention and any other associated losses and (2) either the animal (or herd) value associated with successful intervention or (3) the animal (or herd) value associated with unsuccessful intervention.

A monetary outcome is not always automatically at its correct amount for subsequent comparison with the monetary outcomes determined for the other branches of the decision tree. A monetary value is at its correct amount if the branch of the decision tree to which it is assigned traces directly back from the terminal node to the original decision node without coursing through a chance node. It is not at its correct amount if it was assigned to a branch of a decision tree that traced back from the terminal node through one or more chance nodes, with associated probabilities, to the original decision node. In the latter situation, to determine the correct amount, begin by multiplying the monetary value of the outcome at the terminal node by the probability associated with the branch leading from this node back to the first chance node that it connects to. Repeat this procedure for the remaining monetary values assigned to terminal nodes with branches leading back to this same chance node. These new "weighted" monetary values are then summed to provide a revised monetary value at the level of this chance node. Continue repeating this procedure for each of the other monetary outcomes with branches involving chance nodes, progressively working from right to left until all branches of the decision tree have been collapsed down to only the decisions that emanate from the original decision node and a single "weighted" monetary value is associated with each. These monetary outcomes are then compared against each other and only one is ultimately selected that satisfies the predetermined selection criteria of the decision maker.

The scenario at right illustrates the decision tree concept: As the veterinarian, must help a producer make a decision whether to treat a purebred beef cow with a foot injury of traumatic origin or cull her. Because of her pedigree, she is worth $2,000. Her slaughter value is $450. The diagnostic work-up showed arthritic changes, involving the distal interphalangeal joint of the right front foot. Sepsis is likely present (small draining tract is present). If a claw amputation is performed, based on clinical experience, you estimate that 90% of your surgeries are successful. The cost of surgery and aftercare is $1,000. The cow will be worth the aforementioned $2,000 if she recovers fully (i.e., can ambulate again without limping). If surgical treatment is unsuccessful, the cow can still be culled for $450. You estimate that 25% of cows treated medically with antibiotics and other supportive care recover fully (medical cost = $600). If medical therapy fails, the cow can still be culled for $450, but only if antibiotic residues are not present in the meat (95% chance). Otherwise, the slaughter value is lost.

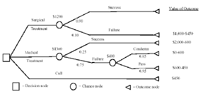
Decision tree concept

The decision tree tells you that, given the aforementioned economic values and probabilities, the expected value for this bull following surgical intervention is $1290, i.e., ([0.85] X [$2500 - 985]) + ([0.15] X [$1000 - 985]), and $1360, i.e., ([0.60] X [$2500 - 500]) + ([0.40] X [0.10 X ($0-500)]) + ([0.90] X [$1000 - 500]), following medical treatment. If no intervention is chosen, the value of the bull is its cull (slaughter) value of $1000. Because this owner is interested in maximizing the value of the bull, the decision tree indicates that medical treatment should be the intervention of choice ($1360 > $1290 > $1000).

A decision tree can also be used to show what the expected value of the bull must be in order for a decision of pursuing medical or surgical intervention to be equivocal. This monetary point (breakeven point) equals the difference between surgical (C1) and medical (C2) treatment cost divided by the difference in the probability of a favorable outcome for surgical (P1) and medical (P2) treatment:

Breakeven (BE) = (C1-C2)/(P1-P2), where C1 = $985, C2 = $500, P1 = 0.85, P2 = 0.60

BE= ($985-$500)/(0.85-0.60) = $1940

Long-Term Decision Making

A longer-term perspective (>1 year) is typically the focus when capital budgeting decisions are being made. Capital budgeting is the process of choosing an investment project based on a comparison of the initial investment cost of the asset with its expected future cash flows when put to use.7 Central to a long-term decision is whether investing in the initial cost of the asset will outweigh the expected cash flow generated from its use. In other words, instead of investing in this asset, should the money be used for something else?

Numerous techniques have been employed to help answer this question including payback period, internal rate of return, book rate of return, and net present value (NPV). The latter technique leads to better investment decisions than the other three methods because it takes into account the time value of money. Hence NPV is discussed next.

Net Present Value

The NPV concept recognizes that a quantity of money received sometime in the future is worth less than the same amount of money received today. Alternatively, more money must be received in the future to equal the same amount of money today. But how much more? The answer is dependent on the discount (interest) rate chosen by the holder of this money.

The formula for NPV is:

The formula takes into account (1) the initial outflow of cash (purchase price of asset and associated costs to put it into use), (2) yearly net cash inflows over the period of time under consideration, and (3) a discount rate. The discount rate should reflect a reasonable return on the monetary commitment. For production agriculture, a discount rate of 8% has been used historically for a long-term investment.

Click formula to enlarge

The NPV value is determined by summing the discounted cash inflows obtained for each year and subtracting this dollar amount from the initial outflow of cash used to purchase and put the asset into service. Consistent with discounted cash flow, the further out in time that a series of net cash inflows goes, the lower the amount each contributes to total cash inflow.

The investment opportunity is deemed acceptable if NPV is greater than $0, whereas it should be rejected if NPV is less than $0. Indifference to the investment decision occurs if NPV equals $0. If several different scenarios are under consideration, the one with the highest NPV should be pursued.

The following example illustrates the use of NPV methodology: A rancher is offered a parcel of grazing land for $220,000 (200 acres @ $1100/acre), which he can pay for with his own money. The only intended use of the land will be to lease it out for contract grazing over a 6-year period. The lessee's annual grazing fee will be $5000 ($25/acre/yr) to be paid at the end of each year. The tax obligation to the rancher (lessor) for the revenue received for each year of this lease is 28%. The lessee has responsibility for all maintenance of the property. At the end of the contract grazing period, the rancher can resell the property to another rancher for $1300/acre. Capital gains on the sale of the land are 15%. The discount rate is 8%. Inflation is not considered.

Is the investment in this parcel of land a good deal for its intended use? Without considering the time value of money, this investment appears to be a good deal because pretax net cash inflow is $64,000 (6 years @ $5,000/yr lease income plus $34,000 appreciation in land value at time of resale) or $55,600 after-tax net cash inflow (6 years @ $3,600* /year net income from leasing the land plus $34,000** net income from resale of the land). However, when the time value of money is considered, the net inflow of cash coming from this land investment is $43,295 less than the original amount of money used to purchase it:

NPV = – $220,000 + $3600*/1.081 + $3600/1.082 + $3600/1.083 + $3600/1.084 + $3600/1.085 + $3600/1.086 + $254,000**/1.086

= –$220,000 + ($3,333 + $3,086 + $2,858 + $2,646 + $2,450 + $2,269 + $160,063)

= –$220,000 + $176,705

= –$43,295

*$5000 – ($5000 x .28) = $3600

**($1300/ac x 200ac) – {[($1300/ac x 200ac) – ($1100/ac x 200ac] x 15} = $254,000

Because NPV is negative, the land purchase price is not a good deal at $1000/acre under the current set of expected net cash inflows coming from its intended use.

Reference

Kasari TR. Economic Analysis Techniques for the Cow-Calf Practitioner. In: Anderson DE and Rings M, eds. Current Veterinary Therapy-Food Animal Practice. 5th ed. Philadelphia: WB Saunders Co. 2008, in press.