Too much of a good thing: Update on NSAID therapy (Proceedings)
The principles of pharmacology upon which a dosing regimen is based, and the impact of disease in the critical patient were discussed in the companion manuscript entitled "Adjusting Doses" in this same proceedings.
The principles of pharmacology upon which a dosing regimen is based, and the impact of disease in the critical patient were discussed in the companion manuscript entitled "Adjusting Doses" in this same proceedings.
Dosing regimens: the PK-PD Dance. Dosing regimens are comprised of a route, dose and interval and are designed to achieve a plasma drug concentration (PDC) within a targeted therapeutic range. In general, drugs are characterized by a dose –concentration- response relationship which indicates that a change in the first results in a comparable and like change in the next. Integrated PK/PD modeling quantitatively describes this relationship by linking the concentration-time course resulting from a dosing regimen (pharmacokinetics [PK]) to the intensity of the observed (and ideally, desired) response to PDC as quantified by pharmacodynamics (PD). Whereas PK relationships are generally linear at clinically-relevant doses, PD-PDC relationships are not: generally a maximum response will be realized beyond which further drug exposure will not increase. For some drugs, the dose best describes the relationship, for others, the concentration. However, concentration-response relationships may be poorly defined for selected drugs, often perhaps for reasons not yet identified (eg, pharmacogenomic differences among species, presence of active but not detected metabolites, enantiomers, saturation kinetics [a shift from first order to zero order kinetics]). The therapeutic range is comprised of a Cmax, above which side effects (adverse drug events [ADE] manifested as exaggerated primary or secondary responses to the drug) or toxicity (ADE characterized by cytotoxicity and associated organ damage), and Cmin, below which therapeutic failure (also an ADE) may occur. Note that the therapeutic range is a population statistic that describes the concentrations between which most animals will exhibit the desired pharmacologic response when given the drug of interest. However, a proportion of the population will respond above and below that range. For those patients, failure should not be considered simply because the patient exceeded the Cmax, unless toxicity occurs. On the other hand, if the patient has not responded once Cmax, has been exceeded, then further dose increase is less likely to achieve the desired response and an alternative (or additional) therapy might be considered. Likewise, drug therapy (eg, anticonvulsants) should not be considered unnecessary if a patient responds below Cmin. Therapeutic drug monitoring should be considered whenever possible to establish the therapeutic range for the critical patient (CP) receiving critical drugs and to remove the trial and error approach to dose modification. Many therapeutic ranges targeted in companion animals are based on extrapolations from human (an exception might be minimum inhibitory concentrations [MIC]) determined on isolates infecting patients and selected cardiac and anticonvulsant drugs. As such, care should be taken with direct extrapolation and when such extrapolations are made, the scientific veterinary community should strive to confirm their relevance.
The route selected for the CP may be limited by drug availability and patient tolerance/client convenience. For the CP, IV is often preferred, in part, to assure 100% drug delivery. Both dose [D] and interval [T] are determined by both pharmacodynamics (PD) and pharmacokinetics (PK). The relationship between PK and PD differs with the drug. For example, for some drugs, the desired response is best achieved if a maximum drug concentration (Cmax) achieved; the duration of exposure is less important. Drugs which irreversibly impair a target might exhibit such a PK-PD relationship: aspirin, which irreversibly acetylates platelets will remain effective until new platelets are formed; aminoglycosides which irreversibly impair bacterial ribosomal activity and proton-pump inhibitors which irreversibly inhibit H+/K+ transport. Drugs tightly adherent to receptors may also fall under this category. For such drugs, duration of effect reflects recovery of the target tissue more than the continued presence of the drug (and thus drug half-life). For other drugs, duration of effect depends on the continued presence of the drug; as such, the dose can be designed to simply remain above a Cmin as long as PDC persist above this target for most of the dosing interval. Drugs which reversibly interfere with receptors often fall into this category: eg, NSAID effects on COX-2, time dependent antibiotics, anticonvulsants, many cardiac drugs. For such drugs, assuming the dose is sufficient to induce the desire response, the interval is important and generally is based on drug half-life (see below).
Dose: Once a targeted PDC is identified (eg Cmax, Cmin or Caverage at steady-state [Css]), determination of the dose depends upon whether or not the drug will be given more than once, and whether or not the drug will accumulate. For drugs administered once, or more than once but at an interval such that the drug does not accumulate (see below), the IV dose (which assures 100% of drug enters circulation) is based on the tissue that will dilute the drug, that is, the apparent volume of distribution (Vd). Thus, Dose = Vd * targeted PDC. For extravascular routes, the dose must be adjusted for less than 100% bioavailability: Dose = Vd* targeted PDC/F (F=fraction of dose absorbed). Thus, in a drug naïve dog suffering from cluster seizures, the IV dose for Phenobarbital (Vd=0.6 L/kg) necessary to achieve the minimum therapeutic range recommended in dogs (15 to 20 mcg/ml [mg/l]) is 0.6L/kg*15 mg/L = 9 mg/kg. This would also be the loading dose to be given for multiple dosing (see below). Phenobarbital is 100% bioavailable, thus the oral dose = the IV dose.
Like dose, if dosing more than once, the choice of interval (T) is influenced by related PK and PD factors and is determined by how much fluctuation will be tolerated during a dosing interval (Cmax - Cmin )and the time that can elapse before Cmax declines to Cmin. The time that can elapse depends on the rate that drug is eliminated from the plasma, or the elimination rate constant (kel). This PK term describes the fraction (which is a "first" order term, rather than amount which is a "zero" order term) of drug removed from the blood per unit time. It is mathematically represented by the slope (rise/run) of the line that describes the time (linear) versus concentration (log) curve (semilogarithmic) of a drug. Elimination rate constants can be obtained from the literature, package inserts, or from two time points (ie, monitoring) of drug concentrations collected during the same dosing interval: C1, T1 and C2, T2. For example, assume cyclosporine (CsA), 2 hr peak (t=2 h) and 12 hr trough (t=12 h) concentrations are 863 and 125 ng/ml, respectively. The kel is the rise/run of the line between these two data points, that is, C1-C2 /T2-T1 . However, because concentration is logarithmic while time is linear, the equation becomes kel= ln[C1/C2]/T2-T1 where ln is the natural log. For this example, the kel for CsA in this patient would be ln[863/125]/12-2, or 0.19 hr-1, indicating 19% of the drug remaining in the body is eliminated each hr. The utility of kel emerges if the targeted Cmax and Cmin are known. For example, assume the dose is redesigned such that the recommended peak (2 h) concentration of 1400 ng/ml is reached. Using the dog Vd of 8 l/kg, Dose = 8 l/kg*1400 ng/ml [1.4 mcg/mL or 1.4 mg/L] = 11.2 mg/kg (this means that the recommended dose of 5 mg/kg probably only achieves 700 ng/ml following a single dose). How long can the dosing interval (T [or T2-T1]) be in this patient before the (lowest) recommended trough of 400 ng/ml is reached? If C1 = 1400 ng/ml and C2 = 400 ng, then T (or T2-T1) = ln[1400/600]/ kel or ln[1400/400]/ 0.19 hr-1, = 1.257/0.19 or 6.6 hr. Thus, an 8 hr dosing interval would be preferred to an 12 hr dosing interval using these targets. A more easily understood measure of elimination is the plasma elimination half-life (t1/2), which is the time needed for 50% of in the blood to be eliminated (again, either by distribution or excretion). The half-life can be determined directly off of a plot of as few as two data points (when plotted semi logarithmically). However, it also can be easily calculated from kel when T2-T1 is defined as a drug half-life: if kel= ln[C1/C2]/T2-T1, then kel= ln[C1/C2]/t1/2 or t1/2 = ln[C1/C2]/ kel. By definition, at one half-life, the relationship between C1 and C2 becomes 2 (that is, C2 is half of C1), and because the ln of 2 = 0.693, then t1/2 = ln[C1/C2]/ kel or kel = 0.693/ t1/2. For monitoring purposes, kel.or t1/2 can be determined from two points: if CsA is 959 at 2 hr and 150 ng/ml at 12 hr, kel = ln (959/150)/ (12-2h) = 1.85/10h = 0.1845. Thus, t1/2 = 0.693/0.1845= 3.75 hr. For this patient, even an 8 hr dosing interval is too long to maintain drug concentrations within the therapeutic range.
Does this make sense? Even without calculators, based on the peak and trough concentrations in this patient, one can "guestimate" that 3 drug half-lives have lapsed during the 10 hr period between samples: 950 (ng/ml) to 475 to 237 to 120 (approximate). Thus, a guestimate half-life would be 10/3 or 3.3 hr. As a general rule of thumb, if the maximum allowable ratio of Cmax:Cmin during the dosing interval is 2, the duration of the dosing interval can be one half-life. If the maximum allowable change is 4, the maximum duration is 2 half-lives (a ratio of 8 will allow 3 half-lives, of 16, 4 half-lives, etc).
Accumulation occurs whenever the dosing interval of the drug is shorter than 3-5 drug elimination half-lives (T<< 3-5*t1/2), that is, not all of the drug given at the beginning of the dosing interval is eliminated before the next dose. As such, drug will accumulate with each dose until steady-state (Css) is reached, that is, tthe amount of drug cleared equals the amount of drug entering the body. The time to steady-state is 3 to 5 drug half-lives of the drug after administration of the same dose. Every time the dose is changed (increased, decreased or discontinued), the time to steady-state begins again and the maximum effect of the dose change should not be assessed until that time. With any dosing regimen, the amount of drug that will have accumulated at steady-state varies with the relationship between the drug elimination half-life (t1/2) and dosing interval (T). The shorter T is compared to t1/2, the less time for drug to be eliminated during a given dosing interval, and the more remains before the next dose is administered and the greater the proportion of each dose that remains in the body. The accumulation ratio (R) describes the relationship of Cmax (or Cmin) achieved at the first dose to Cmax (or Cmin) after administration of a dose at steady-state. The ratio greater the magnitude between t1/2 and T, the greater the magnitude of accumulation. The ratio can be mathematically determined: R = 1/1-0.5z (or R=1/(1-e-kel * T) where z = T/ t1/2 , e is the base of natural logarithm (eg, approximately 2.72), kel is the elimination rate constant or 0.693/ t1/2), T is the dosing interval. Accumulation can also be guestimated by comparing T to t1/2: For example, a drug that is administered every half-life will accumulate two-fold (Css = 2*Cmax); if administered every two half-lives, Css will be approximately 33% higher than Cmax following the first dose; every three half-lives, accumulation will approximate 12.5%. On the other hand, a drug that is administered at an interval that is 50% shorter than the drug half-life will accumulate 3 fold, 25% shorter (6 fold), 12.5% shorter, 12 fold etc. For example, using a 12 hr dosing interval for bromide (21 day half-life in dogs), an accumulation ratio of 63 fold can be expected!
For drugs administered at a half-life that is longer than the dosing interval (T>> t1/2 ), the fluctuation between Cmax to Cmin fluctuation during the dosing interval is progressively larger as T exceeds t1/2 . For such drugs, the concept of steady-state is misleading. Because most drug is eliminated, a true steady-state does not occur. Cmax and Cmin do not fluctuate between but do fluctuate within a dosing interval, thus equilibrium is not truly reached in tissues. The magnitude of fluctuation (like the magnitude of accumulation) depends on the half-life: fluctuation is 87.5% 75% and 50% during the dosing interval if a drug is administered every 3, 2 or 1 half-lives, respectively. The amount of fluctuation, or the relationship (ratio) between Cmax and Cmin can be calculated for a given interval (T): ln[Cmax/Cmin] = T/ kel (where kel = 0.693/t1/2). For some drugs, fluctuation is desirable (ie, concentration antibiotics) or of little concern (drugs which irreversibly bind to receptors). For other drugs, fluctuation must be kept to a minimum. Generally, avoiding more than 50% fluctuation is a reasonable target, thus drugs often are given every half-life or less. The clinician administering a drug with a short-half-life and characterized by time dependency is faced with a tough decision: either administer the drug at an unreasonably short interval, or increase the dose such that the dosing interval can be prolonged. Remembering that each doubling of the dose only "buys" one half-life, increasing the dose until an acceptable interval is reached may result in unacceptably high Cmax and low Cmin. Slow release preparations (ie transdermal patches) provide constant drug input during the dosing interval, thus avoiding both an unreasonable dosing interval and inappropriate fluctuation in PDC. However, for IV preparations, such drugs can be administered as a constant rate infusion (CRI). Constant rate infusions enhance compliance by avoiding untenably short dosing intervals, or enhance drug safety and efficacy by minimizing an undesirable fluctuation between Cmax and Cmin that would occur with a more reasonable dosing intervals. At steady-state, with CRI, Cmax = Cmin = Caverage as long as the drug is being administered at the same rate. As with drugs with a long-half-life given at a short dosing interval, understanding CRI requires understanding the principle of accumulation. Simplistically, CRI involves administration of a drug at an (infinitely small) interval that is shorter than the drug half-life. The rate of infusion (Ro) necessary to achieve a targeted steady-state plasma drug concentration (Css ) depends drug CL; at steady-state, by definition, the amount of drug going into the patient should equal the amount of drug leaving (irreversibly) the patient. Thus, Ro=Css*CL. Clearance, in turn, the volume of blood from which drug is eliminated; the relationship CL = Vd* kel (Ro=Css*Vd* kel). kel can be calculated from half-life (Ro=Css*Vd*0.693/t1/2 ). With CRI, because the dosing interval is infinitely small, the magnitude of accumulation is essentially based on the half-life of the drug (which in turn, depends on clearance and volume of distribution; see companion article). The Css can be predicted by the same equation, that is, the ratio of the rate of infusion (Ro; eg, mg/kg/min) and plasma clearance (CL; ml/kg/min): Css = CL/ Ro. Note that both CL and Vd are pharmacokinetic parameters that must be determined following IV administration to assure that 100% of the dose enters the plasma (an exception for clearance occurs only if the appearance of the drug or its metabolite has been measured in the organ of excretion). Thus, if searching the literature for canine, feline or equine parameters to use for calculations, terms such as Vd/F and CL/F, or data from non-IV studies is not acceptable unless corrected for less than 100% bioavailability.
As with any dosing regimen, the time to reach steady-state concentrations with an infusion is dependent on drug half-life (3-5 half-lives). Although therapeutic concentrations may occur before steady-state is reached, the maximum effect will not occur until that point. For many critical patients, health or well being may be put at risk while waiting for maximum therapeutic response. In such situations, a loading dose can be given (as may occur when administered any drug with a long half-life, regardless of the route). Note, however, that the loading dose is designed to immediately achieve concentrations that will occur at steady-state; however, the patient is NOT at steady-state and will not be until the same dose is administered for 3-5 half-lives. Thus, a loading dose is always accompanied by a maintenance dose that is designed to maintain what the loading dose achieved while steady-state is being reached. Ideally, the sum of drug concentrations declining from the loading dose (50% eliminated each half-life) and the drug concentrations increasing from the maintenance dose (50% accumulated each half-life) should equal Css. However, if the maintenance dose does not maintain what the loading dose achieved, PDC may become toxic or subtherapeutic as steady-state is reached. The loading dose for any drug, whether administered as IV bolus or CRI, is calculated based on the targeted concentration (eg, Cmax, Cmin Cavg, or Css.) and the volume to which the drug is distributed. Thus, the loading dose (DL) = Css. * Vd. The difference between the loading and maintenance doses depends upon the drug half-life: the longer the half-life, the longer to steady-state and the greater the accumulation.
Other considerations regarding CRI include the following: 1. Distribution, not clearance, impacts the concentration achieved with a loading dose (Dose = Vd*Css).. Thus, modification of a loading dose should be based on those factors that influence peak plasma drug concentration after administration of the first dose (see Adjusting doses, companion article). Examples include obesity (reduce dose of water soluble drug), overhydration (increase dose of water soluble drug), dehydration (rehydrate, but if necessary, approach as with altered circulation), altered circulation (decrease dose of drugs whose side effects are manifested in the heart and brain). In general, the loading dose of highly-protein bound drugs does not need to be altered unless drug clearance is impacted by patient health. 2. Administration of a drug by CRI DOES NOT allow rapid manipulation of PDC. 2. Distribution and clearance impacts the maintenance dose. 3. Any time the dose (Ro) is changed, a new steady-state is reached only at 3-5 half-lives. Thus, the duration to response may be rapid (catecholamine vasopressors, half-lives 2 min, time to steady-state [or drug elimination] = 6 minutes) or longer (fentanyl, half-life 2 to 6 hr, time to maximum effect [including drug elimination] 6 to 30 hr). 4 If the drug is characterized by active or toxic metabolites, note that the total area under the curve of the metabolites can surpass the parent compound and that the half-life of the metabolites is often longer than the parent compound. Thus, the time to steady-state, and the maximum effect achieved at steady-state, must also take into account active (toxic) metabolites. Indications for administration of a drug by CRI focus principally on drug half-life and the need to design a convenient dosing interval. The shorter the half-life, and the more narrow the ideal Cmax: Cmin, the greater the need for CRI. Thus, a drug with a short half-life need not necessarily be administered by CRI if it is sufficiently safe that the dose can be increased high enough to extend the dosing interval (ie, high therapeutic index), or if the mechanism of action of the drug is not sensitive to fluctuations in Cmax : Cmin.
Calculators: Several Constant Rate Infusion Calculators are available on the internet. These include: (1) http://www.vin.com/Members/Calculators/calc.plx?CalcID=7. However, this calculator assumes that the dose is already known and provides guidance in building the fluid based on the concentration of the preparation. Likewise, for non vin members, http://www.vasg.org/resources_&_support_material.htm offers rates for a number of analgesic drugs based on a chosen dose. Neither of these calculators provide information on the plasma concentration that will be achieved. If a concentration is known, http://www.chm.davidson.edu/erstevens/stevens_med.html can be used to help determine what dose is needed to get there. It is best understood with a little background tutoring in pharmacokinetics, but it offer options for comparing administration of a drug as an IV bolus versus and IV infusion with or without a loading dose. As such, information required for assessing the CRI includes Dose (loading and maintenance), elimination rate constant (0.693/half-life), volume of distribution and planned time of infusion. This site also demonstrates drug concentrations after the infusion has stopped.
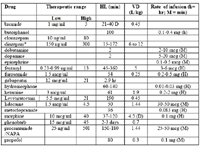
Constant rate infusion amounts
Examples: Analgesics: In general, response to opioid analgesics is dependent on PDC. An exception occurs for drugs such as buprenorphine which adheres tightly to receptors and thus may be effective even in the presence of very low PDC. In one study in normal dogs, the Vd of fentanyl was 10.65 ± 5.53 l/kg and its t1/2 ranged from 2.5 to 6 hr. Using 4 hr as the t1/2, then kel = 0.693/4 = 0.173 hr-1 and CL becomes (0.173 hr-1 * 10.65 L/kg) = 1.845 L/kg/hr. To target Cmin or 1 ng/ml of fentanyl at steady-state (12-20 hr), the rate of infusion Ro = ([1 ng/ml] * 1845 ml/kg/hr) = 1845 ng/kg/hr (1.845 mcg/kg/hr or 0.242 mcg/kg/min). Steady-state should be reached in approximately 12 to 20 hrs. If this is too long, to immediately target 1 ng/ml (Cmin), a loading dose (DL = Css*Vd).of (10.5 L/kg * 1 mcg/L) or 10.5 mcg/kg would be administered IV. To target Cmax (ie, 13 ng/ml) at steady-state, Ro (maintenance dose) = 13.5 ng/nl*1845 mg/kg/hr = 23895 ng/kg/hr (23 mcg/kg/hr or 0.383 mcg/kg/min); the DL = 13 mcg/L*10.5L/kg = 135 mcg/kg. Note the ratio of DL to Ro = 135:23. An approach that might be more prudent than targeting either Cmin or Cmax is targeting a Css that is an average of the two extremes. Additionally, the DL and Ro do not have to "match" exactly as long as the clinician is aware of the PDC targeted by each dose. For morphine, its half-life is shorter and as such, the relationship between the loading and maintenance dose should be smaller that it is for fentanyl. For morphine, to target 10 mcg/ml at steady-state (1.5 h) = Ro = [10 ng/ml] *CL. Clearance is not available, so it will be calculated from CL=Vd*kel where kel = 0.693/t1/2 = 0.693/1.5 hr = 0.462/hr. Thus CL = 4.5 L/kg*0.462/hr = 2.079 L/kg/hr. The rate of infusion = 10 mcg/ml (or 10 mg/L)* 2.079 L/kg/hr = 20.8 mg/kg/hr (or 0.35 mg/kg/min).
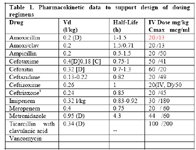
Pharmacokinetic data to support design of dosing regimens
Antiepileptics: The treatment of status epilepticus (SE) in a drug naïve dog can be used to exemplify pharmacokinetic and pharmacodynamic principles of CRI. Three anticonvulsants whose half-life (in normal dogs) is markedly different will be compared: diazepam, Phenobarbital, and bromide (21 days). For diazepam, a target of 200 ng/ml is targeted. The reported half-life ranges from a low of 15 minutes to several hr; 15 minutes appears to be more clinically relevant. Although steady-state will be achieved in about 45 to 60 min, this is too long for a patient in SE, so a loading dose is given: 12 L/kg * 200 mcg/mL = 2400 mcg/kg (2.4 mg/kg). The Ro = 200 mcg/L * 12 L/kg * 0.693/15 min = 110 mcg/kg/min. For Phenobarbital, we will assume that the patient just started dosing at 4 mg/kg and following the first dose, both a peak ( 4 hr; 2.8 mg/L) and trough (12 hr; 2.4 mg/L) sample are collected. From this, a half-life can be calculated: kel is ln[28/24]/12-4 or 0.0192 hr-1; the half-life thus is 0.693/0.0192 hr-1, or 35 hr. Steady-state will not occur until approximately 105 hr (4 days). If a 12 hr dosing interval is used, predicted steady-state peak concentrations would be 2.8 mg/L * R where R = 1/1- e-0.0192 * 12 or 1/1-0.83 = 1/0.1.7 = 5.79*2.8 or 16.2 mcg/ml. The accumulation ratio in this patient would be 16.2: 2.8 or about 6. If the patient is in status epilepticus, a loading dose of 0.7 L/kg* 16 mg/L or 11.2 mg/kg could be given IV to immediately achieve steady-state concentrations. The long half-life of Phenobarbital precludes the need for CRI. For bromide, with a 21 day half-life, as demonstrated above, the accumulation ratio is 63. To target the low end of the therapeutic range with bromide, a dose of 1 mg/ml (1000 mg/L) * 0.45 L/kg or 450 mg/kg should be administered. This compares to a daily maintenance dose of 30 mg/kg to maintain 1 mg/ml.
Antibiotics: Time dependent antimicrobials are most effective if drug concentrations (plasma or tissue) remain above the minimum inhibitory concentration (MIC) of the infecting microbe for the majority of the dosing interval. The minimum duration is 50-75%, with 75-100% desirable to prevent resistance. The accepted dosing interval depends upon the distance between the plasma (tissue) antimicrobial concentration achieved with dosing and the infecting microbe. For each log magnitude of distance between the two, one elimination half-life can lapse before PDC=MIC. For example, assume the MIC for E. coli for ampicillin (and amoxicillin) 8 mcg/ml (breakpoint MIC = 32 mcg/ml, indicating a susceptible organism) and for amoxicillin-clavulanic acid, 2 mcg/ml. Using Table 1, the Cmax:MIC at each at (dose mg/kg) for three dosing options are IV ampicillin (50:8 [6.25] @20 mg/kg), SC ampicillin (14:8 [1.75] at 12 mg/kg), and oral amoxicillin-clavulanic acid (11.4:8 [1.4]at 17 mg/kg). The number of half-lives that lapse before PDC reach the MIC for each drug at the dose are between 2-3 for ampicillin IV (between 2*2 [4] and 2*2*2 [8]), and less than 1 for either ampicillin SC or amoxicillin-clavulanic acid (thus, neither of these two choices is acceptable). Alternatively, at 50 mcg/ml, in one half-life (1HL) drug concentrations have decreased to 25, by 2 HL to 12.5, by 3 HL to 6.25. The half-life for ampicillin is between 0.5 and 1.5 hr (1 h). Thus, between 2-3 h can lapse before PDC=MIC. If this represents 50% of the dosing interval, then a 4 to 6 hr dosing interval for ampicillin is acceptable as long as the following assumptions hold true: 1. all drug in plasma reaches the microbe (a potentially safe assumption only if infection is located in uncomplicated tissues characterized by fenestrated capillaries); 2. avoiding resistance is not the goal. If the latter is the goal, only a 2-3 hr interval is appropriate. If only 30% of drug penetrates infecting tissue, the dosing interval should be at most 1 hr. Clearly, constant rate infusion is a viable alternative for treatment of this infection. To target an ampicillin Css of 8 mcg/ml, the ROI = Css* CL. CL is not available, but it can be calculated from Vd*Kel where kel = 0.693/HL (0.693/1h = 0.693/h). Thus CL = 0.2 L/kg * 0.693/hr = 0.1386 L/hr/kg. The ROI becomes 0.13h6 L/h/kg*8 mcg/ml (8 mg/L) or 1.11 mg/kg/hr. The patient reaches steady-state in 3 to 5 hr. The need for a loading dose is questionable, in part based on the mechanism of action of beta-lactams. Further, the risk of endotoxin release might indicate a gradual climb to steady-state is more appropriate. However, if a loading dose is given, 0.2L/kg * 8mg/L = 1.6 mg/kg.
Podcast CE: A Surgeon’s Perspective on Current Trends for the Management of Osteoarthritis, Part 1
May 17th 2024David L. Dycus, DVM, MS, CCRP, DACVS joins Adam Christman, DVM, MBA, to discuss a proactive approach to the diagnosis of osteoarthritis and the best tools for general practice.
Listen