Using epidemiologic principles to conduct a disease investigation (Proceedings)

When planning a disease outbreak investigation, it is very helpful to know beforehand the major risk factors associated with the disease to be investigated. This knowledge can be gained through, for example, reading the scientific literature, conversations with experts, and/or personal experience. A pathway model can be helpful to visualize the interrelationship between risk factors and their effects on each other.

A suggested set of disease investigation steps are presented below. Each is recommended to be conducted in the order given.

Confirm the diagnosis

Physical examination and laboratory findings are critical pieces of data to use to decide if the disease in question is likely to be present.

Develop a case definition

Herd and management data should be collected (e.g. questionnaire) and reviewed to decide what constitutes a case of disease "X". A case could be established based on clinical information, some operational (e.g. treatment response) or statistical (e.g.'normal' within 2 std. dev. of age-specific mean) parameter, or based on some other piece of information.

Find new cases

Surveillance should be changed from a passive nature to one of active surveillance.

Plot epidemic curve

The importance of plotting an epidemic curve is to prove that an epidemic exists, i.e. starting with the index case, determine that a disease is occurring at a level which exceeds its usual frequency (endemic level) over some period of time. Typically, the number of cases of disease is plotted on the Y axis and time of disease occurrence is plotted on the X axis.

The shape of the curve and the time scale depend on the incubation period of the disease, the infectivity of the agent, the proportion of susceptible animals in the population, and the distance between animals (animal density).

Several types of epidemic curves can occur. A common source (point-source) epidemic is one in which all cases are infected from a source that is common to all individuals (e.g. toxin). Typically, all cases of a point-source epidemic occur within one incubation period of the causal agent.

In contrast, a propagating epidemic is one that is caused by an infectious agent in which initial cases (primary or index case) shed the agent and subsequently infect susceptible individuals (secondary cases). The time interval between peaks of successive temporal clusters of cases reflects the incubation period of the causal agent. If the period between subsequent peaks of the epidemic curve is less than the most common incubation period then it is difficult to differentiate between a propagating epidemic and a series of point-source epidemics.

Review cases and non-cases

It is important to view cases and non-cases in a temporal (time) and spatial (place) context.

Formulate a hypothesis about the epidemic

The aforementioned steps should help establish a hypothesis as to the likely disease agent responsible for the disease outbreak in questions. A farm event chart and temporal chart can aid in identifying its mode of transmission, source of infective material, and reservoir host.

A farm events chart is a written record of farm-wide events listed by their date of occurrence, e.g. entry of animals onto premises, feed changes, whole herd vaccination, previous episodes of illness, and any herd-wide management changes. If at all possible, go back at least six months prior to start of the problem. These events should be compared to risk factors from your pathway model.

A temporal chart lists the farm events in chronological order with respect to time. A graph of these events allows juxtaposition of their occurrence with the pattern of disease occurrence (epidemic curve).

Institute temporary control measures

The control measures that are instituted should be based on your working hypothesis for the causative agent (and likely causative risk factors) suspected to be present in the diseased animals. Any or all of these control measures may change as more information is gathered.

Perform a Risk Factor (Case-Control) Analysis

The object of this step is to identify what is different about animals that developed the problem compared with animals that did not develop the problem (but could have). Risk factors from your pathway model should guide which ones to investigate. An epidemiologic analytical technique based on an odds ratio (OR) helps measures strength of the relationship (association) between exposure to risk factors and disease. A 2X2 table summarizing the number of animals in each of four categories is constructed in the manner at left to perform this analysis.

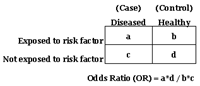
Risk Factor Analysis

The odds ratio represents the odds of disease for animals in the top row of the table relative to the odds of disease for animals in the bottom row. However, the OR gives no indication of its reliability, i.e. how much faith to put in it. An OR of 1.0 implies that there is no association of exposure to the risk factor and disease. An OR >1.0 implies that exposure might be a risk factor. An OR <1.0 implies that the exposure might be a preventive factor.

Tests of statistical significance such as Chi-square test (X2) or confidence interval (CI) can be used to determine how likely it is that the observed OR could have occurred by chance alone, if exposure to risk factor was not actually related to disease. A Chi-square test tells you the probability of finding an association as strong as (or stronger than) the one you have observed if the null hypothesis (i.e. risk factor has no influence on disease occurrence between groups) were really true. At least 20 subjects are needed to perform this test and the expected value in each cell of the 2 X 2 table must be at least 5. A Chi-square larger than 3.84146 corresponds to a p-value smaller than 0.05, i.e. "less than 1 out of 20 chance that the observed association (OR) between the risk factor and diseased animals versus non-diseased animals was due to chance alone". A Chi-square larger than 6.6349 corresponds to a p-value smaller than 0.01, i.e. "less than 1 out of 100 chance that the observed association (OR) between the risk factor and diseased animals versus non-diseased animals was due to chance alone". The following example illustrates the use of the Chi-square test:

Since 0.79363 is less than 3.84146, it is concluded that the risk factor should not be considered further as a causative factor in the epidemiology of the disease in question (Note: the OR is 0.67, which suggests that the risk factor may actually be a preventative (protective) factor).

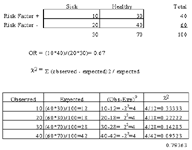
Chi-square test

A confidence interval (CI) is a statistical method that expresses the range over which a value such as OR is likely to occur. A 95% CI means that the probability of including the true value (OR) within the specified range is 0.95. If the CI > 1.0, then it can be concluded that the risk factor is positively associated with disease occurrence. If CI < 1.0, then it can be concluded that the risk factor is negatively associated (protective) with disease occurrence. If CI includes 1.0, then the risk factor is not associated with disease occurrence. The CI is determined as follows:

Since the CI (0.27-1.64) passes through 1.0, it is concluded that the risk factor is not associated with the occurrence of disease.

Confidence interval

Perform risk group-based sampling

Sample at least 10 animals per risk group (animals in the unexposed (control) group; animals in the exposed (case) group).

Update control measures

Document efficacy of control measures using surveillance

Write report

The report should be distributed to all stakeholders.

References

Thrusfield M. Patterns of Disease. In: Thrusfield M, ed. Veterinary Epidemiology. 2nd ed. London: Blackwell Science Ltd. 1995;114-128.

Thrusfield M. Demonstrating Association. In: Thrusfield M, ed. Veterinary Epidemiology. 2nd ed. London: Blackwell Science Ltd. 1995;199-219.

Thrusfield M. Observational Studies. In: Thrusfield M, ed. Veterinary Epidemiology. 2nd ed. London: Blackwell Science Ltd. 1995;220-255.